We have three doors before us. Behind one of them is a car. The game show host invites us to choose a door. We do so, to which he responds by opening a door we have not chosen, revealing a goat. He asks us if we want to stick with our choice or change it. We decide to keep it, thinking we have a 50% chance of success. But we are wrong: our odds would have increased from 1/3 to 2/3 if we had chosen the other door, thanks to the clue the host gave us about the third door by not opening it. This correct conclusion was published by Marilyn vos Savant in 1990 in her column in Parade magazine. The so-called Monty Hall problem—named after the host of the TV game show Let’s Make a Deal, the origin of the three-door problem—is an example of how mathematics can help us make reasoned decisions, something that becomes vital in emergency situations such as a nuclear accident or a pandemic.

But if Savant’s solution seems counterintuitive, contrary to everything we think we know, that’s because it is. So much so that when the columnist—holder of the Guinness World Record for the world’s highest IQ—published her explanation, she received some 10,000 letters of disapproval, a thousand of them signed by mathematicians or PhD holders who went so far as to insult her. In reality, the Monty Hall problem had already been posed and solved in 1975 by the statistician Steve Selvin in the journal The American Statistician, and has an even earlier version in the three-prisoner problem formulated by Martin Gardner in 1959. But it was Savant’s article and the resulting media furore that raised its profile to such an extent that it was endlessly debated, from the Massachusetts Institute of Technology to the Central Intelligence Agency.
So foreign to logic does this idea seem that even the great mathematician Paul Erdös came to deny it, as his friend and colleague Andrew Vazsonyi recounted in his 2002 autobiography Which Door has the Cadillac: Adventures of a Real-Life Mathematician. Vazsonyi recounted how another friend had posed the problem to him, to which his mind immediately reacted with the intuitive answer that it didn’t matter if he changed his choice. Until, he said, the image of the theologian Bayes flashed through his mind.
Bayesian mathematics to save our lives
In the 18th century, the Presbyterian clergyman Thomas Bayes formulated a special case of what we know today as the theorem that bears his name. In its mathematical form, Bayes’ theorem is “an incomprehensible formula that no one could understand, much less use,” in Vazsonyi’s words. However, applied to the real world as Vazsonyi himself liked to do, it becomes a powerful decision-making tool: what Bayes’ theorem comes to describe is how the probability of something happening is altered as we learn more facts. This is rather like learning. In recent times, interest in Bayesian methodology has grown to such an extent that it not only inspires the design of Artificial Intelligence, but some see it as the future of the scientific method; it has even been proposed that the human brain is Bayesian.
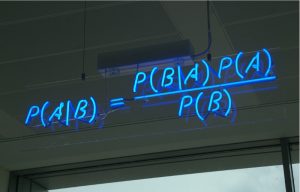
Through Bayes, Vazsonyi found the correct solution to the Monty Hall problem, despite acknowledging that “it makes no sense, but Newton’s law makes no sense either,” he wrote. However, he was surprised that Erdös did not believe its conclusion, knowing that “probability is not a fixed, static thing; it changes as time goes by.” In the end, Erdös was only convinced when Vazsonyi showed him a computer simulation.
In fact, beyond mental brainteasers and TV game shows, it is computer-based mathematical simulations that today dominate the science of decision-making in a multitude of fields, from financial markets to the management of emergencies such as nuclear accidents. And also in the fight against the pandemic: since the emergence of COVID-19, epidemiologists around the world have made extensive use of simulation models to formulate predictions about the evolution of the pandemic in order to aid policy decision making. Some of these models use Bayesian methods.
Looking back, it is fair to say that these models have not always been right, as the experts themselves have acknowledged. But as Bayes teaches us, we get better with learning. And Bayesian mathematics might even save our lives: it helped save fisherman John Aldridge after he fell overboard from his boat in 2013. Since the 1970s, the US Coast Guard has been using Bayesian analysis in its searches for people missing at sea.
Javier Yanes
Comments on this publication